# 0004. 寻找两个正序数组的中位数 ## 题目地址(4. 寻找两个正序数组的中位数) <https://leetcode-cn.com/problems/median-of-two-sorted-arrays/> ## 题目描述 ``` <pre class="calibre18">``` 给定两个大小为 m 和 n 的正序（从小到大）数组 nums1 和 nums2。 请你找出这两个正序数组的中位数，并且要求算法的时间复杂度为 O(log(m + n))。 你可以假设 nums1 和 nums2 不会同时为空。 示例 1: nums1 = [1, 3] nums2 = [2] 则中位数是 2.0 示例 2: nums1 = [1, 2] nums2 = [3, 4] 则中位数是 (2 + 3)/2 = 2.5 ``` ``` ## 前置知识 - 中位数 - 分治法 - 二分查找 ## 公司 - 阿里 - 百度 - 腾讯 ## 思路 首先了解一下 Median 的概念，一个数组中 median 就是把数组分成左右等分的中位数。 如下图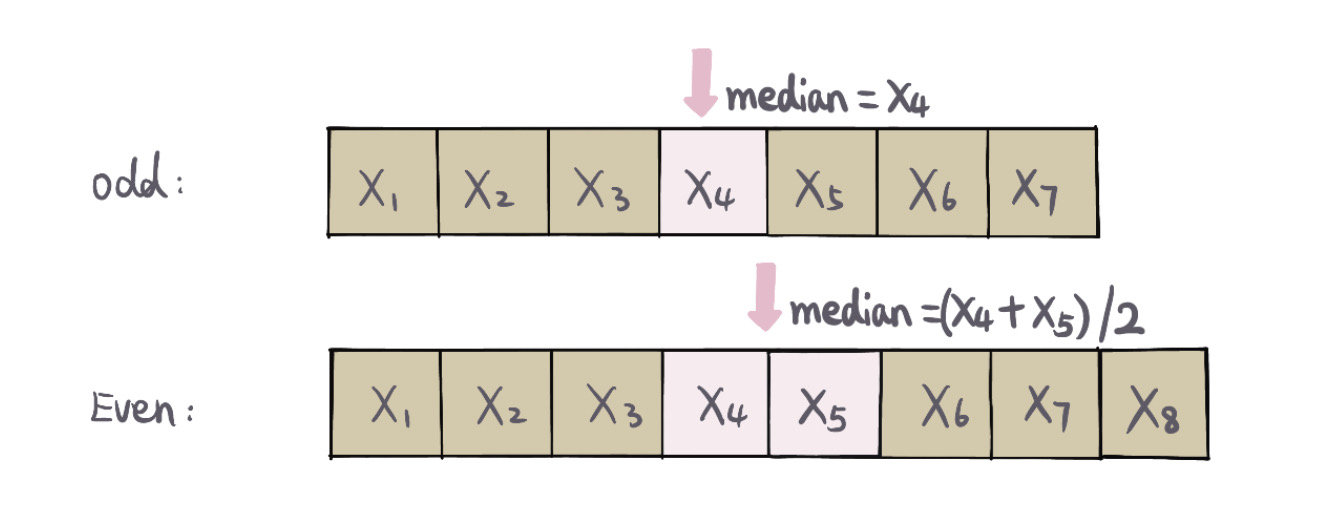：  这道题，很容易想到暴力解法，时间复杂度和空间复杂度都是`O(m+n)`, 不符合题中给出`O(log(m+n))`时间复杂度的要求。 我们可以从简单的解法入手，试了一下，暴力解法也是可以被 Leetcode Accept 的. 分析中会给出两种解法，暴力求解和二分解法。 #### 解法一 - 暴力 （Brute Force） 暴力解主要是要 merge 两个排序的数组`（A，B）`成一个排序的数组。 用两个`pointer（i，j）`，`i` 从数组`A`起始位置开始，即`i=0`开始，`j` 从数组`B`起始位置， 即`j=0`开始. 一一比较 `A[i] 和 B[j]`, 1. 如果`A[i] <= B[j]`, 则把`A[i]` 放入新的数组中，i 往后移一位，即 `i+1`. 2. 如果`A[i] > B[j]`, 则把`B[j]` 放入新的数组中，j 往后移一位，即 `j+1`. 3. 重复步骤#1 和 #2，直到`i`移到`A`最后，或者`j`移到`B`最后。 4. 如果`j`移动到`B`数组最后，那么直接把剩下的所有`A`依次放入新的数组中. 5. 如果`i`移动到`A`数组最后，那么直接把剩下的所有`B`依次放入新的数组中. Merge 的过程如下图。  *时间复杂度： `O(m+n) - m is length of A, n is length of B`* *空间复杂度： `O(m+n)`* #### 解法二 - 二分查找 （Binary Search） 由于题中给出的数组都是排好序的，在排好序的数组中查找很容易想到可以用二分查找（Binary Search), 这里对数组长度小的做二分， 保证数组 A 和 数组 B 做 partition 之后 `len(Aleft)+len(Bleft)=(m+n+1)/2 - m是数组A的长度， n是数组B的长度` 对数组 A 的做 partition 的位置是区间`[0,m]` 如图：  下图给出几种不同情况的例子（注意但左边或者右边没有元素的时候，左边用`INF_MIN`，右边用`INF_MAX`表示左右的元素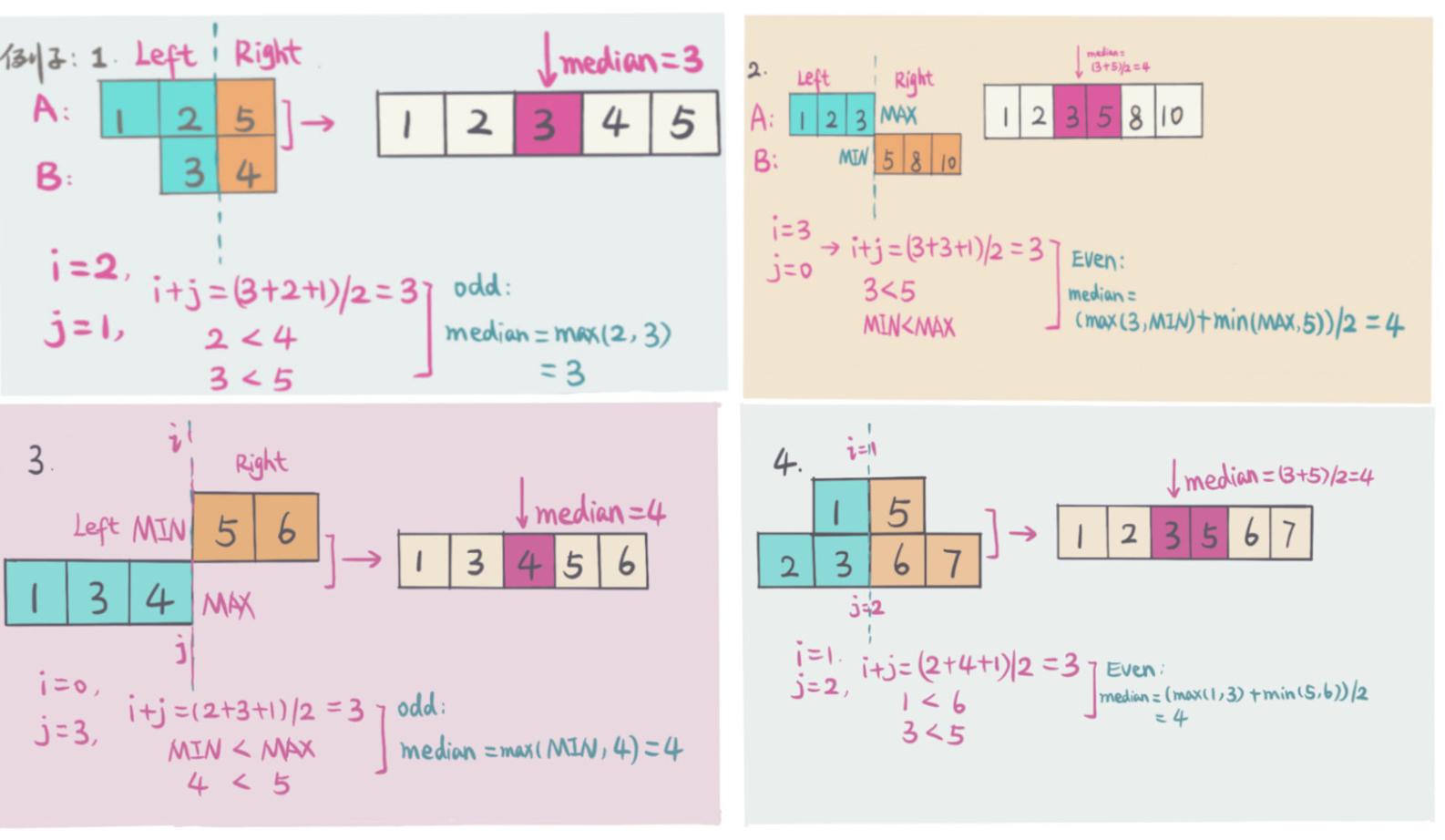：  下图给出具体做的 partition 解题的例子步骤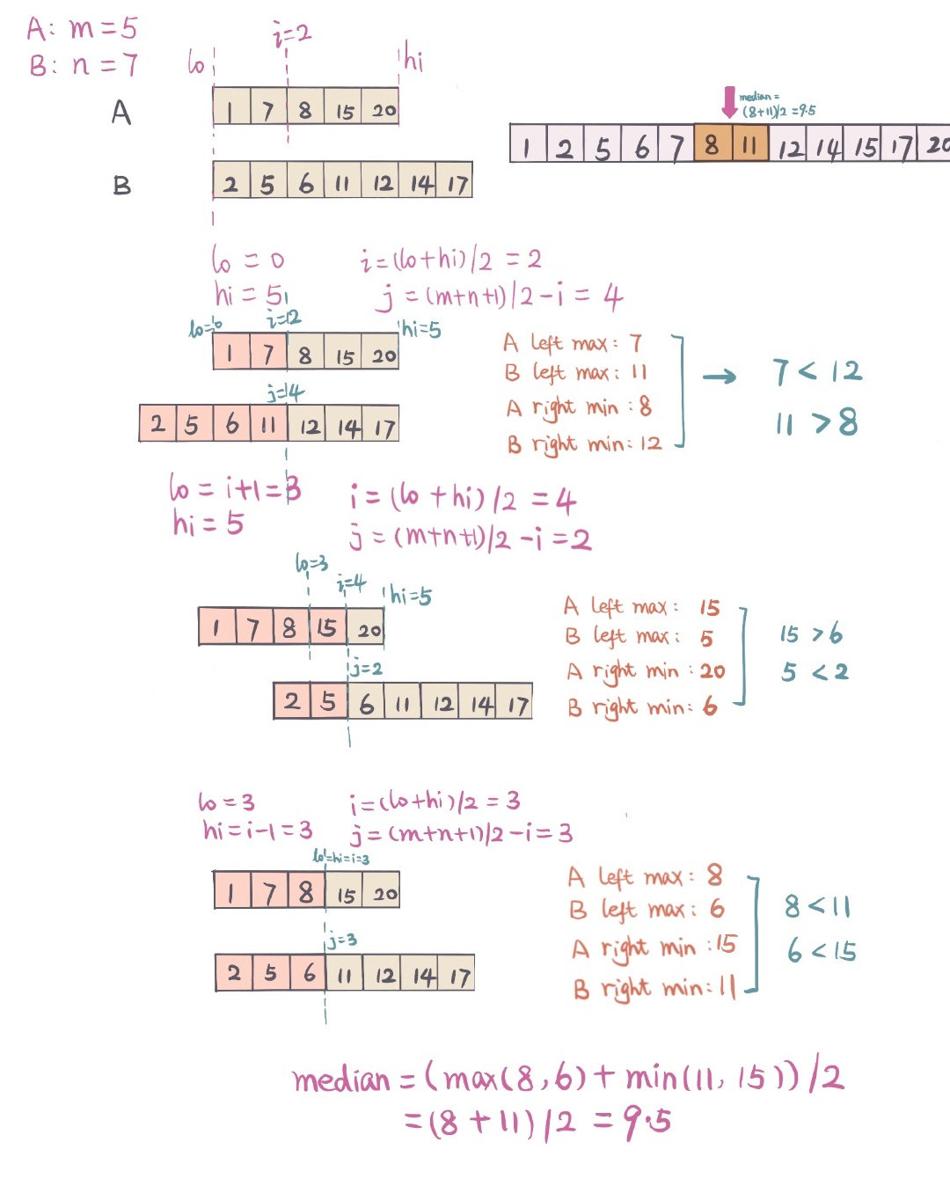，  *时间复杂度： `O(log(min(m, n)) - m is length of A, n is length of B`* *空间复杂度： `O(1)` - 这里没有用额外的空间* ## 关键点分析 1. 暴力求解，在线性时间内 merge 两个排好序的数组成一个数组。 2. 二分查找，关键点在于 3. 要 partition 两个排好序的数组成左右两等份，partition 需要满足`len(Aleft)+len(Bleft)=(m+n+1)/2 - m是数组A的长度， n是数组B的长度` 4. 并且 partition 后 A 左边最大(`maxLeftA`), A 右边最小（`minRightA`), B 左边最大（`maxLeftB`), B 右边最小（`minRightB`) 满足 `(maxLeftA <= minRightB && maxLeftB <= minRightA)` 有了这两个条件，那么 median 就在这四个数中，根据奇数或者是偶数， ``` <pre class="calibre18">``` 奇数： median = max(maxLeftA, maxLeftB) 偶数： median = (max(maxLeftA, maxLeftB) + min(minRightA, minRightB)) / 2 ``` ``` ## 代码 代码支持： Java，JS： Java Code： *解法一 - 暴力解法（Brute force）* ```java \[\] class MedianTwoSortedArrayBruteForce { public double findMedianSortedArrays(int\[\] nums1, int\[\] nums2) { int\[\] newArr = mergeTwoSortedArray(nums1, nums2); int n = newArr.length; if (n % 2 == 0) { // even return (double) (newArr\[n / 2\] + newArr\[n / 2 - 1\]) / 2; } else { // odd return (double) newArr\[n / 2\]; } } private int\[\] mergeTwoSortedArray(int\[\] nums1, int\[\] nums2) { int m = nums1.length; int n = nums2.length; int\[\] res = new int\[m + n\]; int i = 0; int j = 0; int idx = 0; while (i < m && j < n) { if (nums1\[i\] <= nums2\[j\]) { res\[idx++\] = nums1\[i++\]; } else { res\[idx++\] = nums2\[j++\]; } } while (i < m) { res\[idx++\] = nums1\[i++\]; } while (j < n) { res\[idx++\] = nums2\[j++\]; } return res; } } ``` <pre class="calibre18">``` ```javascript [] /** * @param {number[]} nums1 * @param {number[]} nums2 * @return {number} */ var findMedianSortedArrays = function (nums1, nums2) { // 归并排序 const merged = []; let i = 0; let j = 0; while (i < nums1.length && j < nums2.length) { if (nums1[i] < nums2[j]) { merged.push(nums1[i++]); } else { merged.push(nums2[j++]); } } while (i < nums1.length) { merged.push(nums1[i++]); } while (j < nums2.length) { merged.push(nums2[j++]); } const { length } = merged; return length % 2 === 1 ? merged[Math.floor(length / 2)] : (merged[length / 2] + merged[length / 2 - 1]) / 2; }; ``` ``` **复杂度分析** - 时间复杂度：O(max(m,n))O(max(m, n))O(max(m,n)) - 空间复杂度：O(m+n)O(m + n)O(m+n) *解法二 - 二分查找（Binary Search)* ```js \[\] /\*\* - 二分解法 - @param {number\[\]} nums1 - @param {number\[\]} nums2 - @return {number} \*/ var findMedianSortedArrays = function (nums1, nums2) { // make sure to do binary search for shorten array if (nums1.length > nums2.length) { \[nums1, nums2\] = \[nums2, nums1\]; } const m = nums1.length; const n = nums2.length; let low = 0; let high = m; while (low <= high) { const i = low + Math.floor((high - low) / 2); const j = Math.floor((m + n + 1) / 2) - i; const maxLeftA = i === 0 ? -Infinity : nums1\[i - 1\]; const minRightA = i === m ? Infinity : nums1\[i\]; const maxLeftB = j === 0 ? -Infinity : nums2\[j - 1\]; const minRightB = j === n ? Infinity : nums2\[j\]; if (maxLeftA <= minRightB && minRightA >= maxLeftB) { return (m + n) % 2 === 1 ``` <pre class="calibre18">``` ? Math.max(maxLeftA, maxLeftB) : (Math.max(maxLeftA, maxLeftB) + Math.min(minRightA, minRightB)) / 2; ``` ``` } else if (maxLeftA > minRightB) { high = i - 1; } else { low = low + 1; } } }; ``` ```java \[\] class MedianSortedTwoArrayBinarySearch { public static double findMedianSortedArraysBinarySearch(int\[\] nums1, int\[\] nums2) { // do binary search for shorter length array, make sure time complexity log(min(m,n)). if (nums1.length > nums2.length) { return findMedianSortedArraysBinarySearch(nums2, nums1); } int m = nums1.length; int n = nums2.length; int lo = 0; int hi = m; while (lo <= hi) { // partition A position i int i = lo + (hi - lo) / 2; // partition B position j int j = (m + n + 1) / 2 - i; ``` <pre class="calibre18">``` int maxLeftA = i == 0 ? Integer.MIN_VALUE : nums1[i - 1]; int minRightA = i == m ? Integer.MAX_VALUE : nums1[i]; int maxLeftB = j == 0 ? Integer.MIN_VALUE : nums2[j - 1]; int minRightB = j == n ? Integer.MAX_VALUE : nums2[j]; if (maxLeftA <= minRightB && maxLeftB <= minRightA) { // total length is even if ((m + n) % 2 == 0) { return (double) (Math.max(maxLeftA, maxLeftB) + Math.min(minRightA, minRightB)) / 2; } else { // total length is odd return (double) Math.max(maxLeftA, maxLeftB); } } else if (maxLeftA > minRightB) { // binary search left half hi = i - 1; } else { // binary search right half lo = i + 1; } } return 0.0; } ``` ``` } ``` **复杂度分析** - 时间复杂度：O(log(min(m,n)))O(log(min(m, n)))O(log(min(m,n))) - 空间复杂度：O(log(min(m,n)))O(log(min(m, n)))O(log(min(m,n))) 大家对此有何看法，欢迎给我留言，我有时间都会一一查看回答。更多算法套路可以访问我的 LeetCode 题解仓库：<https://github.com/azl397985856/leetcode> 。 目前已经 37K star 啦。 大家也可以关注我的公众号《力扣加加》带你啃下算法这块硬骨头。